It’s been nearly 20 years since Shimano and Hutchinson first introduced tubeless road tires to the world, and more than 25 years since Mavic and Michelin debuted UST on the mountain bike side. Thankfully, the technology has vastly improved since those early days – particularly in terms of tire-and-rim fitment and security – but one particular aspect of tubeless still irks me: why the heck are we still basically guessing when it comes to how much sealant we need to add to our tires?
Horseshoes and hand grenades
If you want to get a recommendation for how much pressure to run in your tubeless tires, there is no shortage of handy online calculators and guides to remove much of the guesswork from the process. Silca was one of the first to offer one when it launched its calculator back in 2014, but SRAM, Wolf Tooth, Enve, Goodyear, and Rene Herse – and almost certainly others I’ve overlooked – have all joined the fray in the days since.
Granted, they don’t all spit out the exact same numbers as each brand has its own nuanced take on what pressures work best for a given situation. However, any of them offer a better starting point than just purely guessing.
Now, say you’re at home (or a mechanic at a shop) installing a fresh set of tubeless tires and you’re wondering how much tubeless sealant you need to add. You know you need enough to fully seal the tire casing and tire-to-rim interface for a reliably airtight setup, you want to have a little extra so there’s enough liquid sealant in reserve to tackle the inevitable puncture or small cut, but you also don’t want to put way more than you need in there (sealant eventually starts getting expensive, after all).
Surely there are similar online calculators to guide you in that process, too, right?
Quite astonishingly, no such thing exists (or at least, nothing that I could find). Further complicating matters, a survey of various sealant brands offer wildly divergent suggestions on how much of their own magic liquid goop they want you to use.
On a 700x40 mm gravel tire, for example, NoTubes is the most specific, recommending 60 mL of sealant for that particular size. Silca says 90-120 mL for a “gravel” tire. Schwalbe says a tire measuring “23-60 mm” should run 60-90 mL. Vittoria only lists “road” and “mountain” tires, and as good as Orange Seal is as a sealant, its guidelines unfortunately aren’t much better.

How about a 29x2.3” mountain bike tire?
Again, NoTubes is the most specific with a recommendation of 105 mL. Orange Seal’s guidelines don’t call out casing width at all, suggesting 3 oz for a 26” or 27.5” tire, and 4 oz for a 29” one. Schwalbe? Probably about 90 mL. Vittoria suggests 80 mL as a starting point for any/all mountain bike tires, regardless of diameter or width.
Clear as mud.
Bikes and donuts: two great tastes that taste great together
There’s an extraordinary breadth of bicycle rim and tire sizes currently in use on roads and trails. While some more progressive road riders might be on 32 mm-wide rubber, others might be on 25s. Modern mountain bikes are more often built around 29” rims with 2.3-2.5”-wide casings, but 27.5” diameters are also common. And gravel? It wasn’t all that long ago that 35 mm casings were considered “high-volume”, but all the cool kids now are on 50s.
Point being, it’s not very useful these days to just refer to “mountain bike” tires or “road” tires or “gravel” tires.
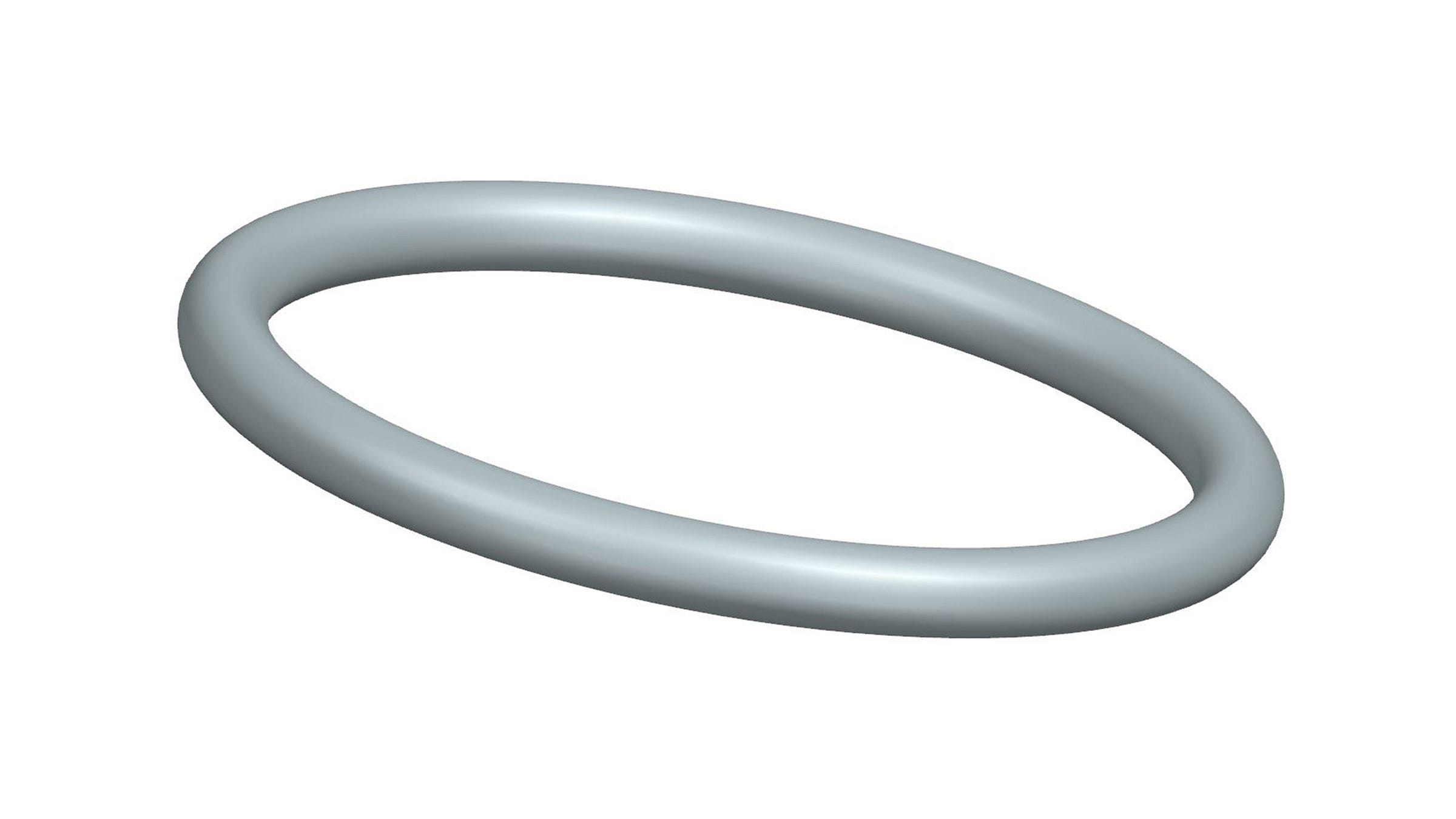
Luckily, regardless of the specific rims and tires used, the resultant shape is always the same: basically a classic donut. In mathematical terms, that donut is more precisely described as a toroid, and conveniently for this application, there are all sorts of established formulas related to that shape.
To figure out a more precise estimate for how much tubeless sealant you need for any given wheel-and-tire combo, you can just take the surface area of that toroid and then multiply it by a desired thickness of sealant throughout the interior. This doesn’t quite reflect reality since sealant isn’t perfectly distributed across the entire interior surface area of the tire and rim, but for our purposes, it nevertheless spits out a useful figure.
To be clear, I’m simplifying things a bit here for the sake of more straightforward calculations (and also because my calculus is very, very, very rusty). Whereas a toroid is technically a cross-section of any shape that’s rotated about an axis in space to produce a ring-like structure, we’re assuming we have a torus here, which has a perfectly circular cross-section (an “ideal” donut, if you will). The cross-section of a bicycle tire-and-rim combo isn’t quite exactly circular, of course, but it’s close enough for our purposes. But by making that assumption, you only need to know two things: your rim diameter and your tire width. That’s it.
There are some unknowns to keep in mind, though.
For example, some tires will require more sealant than others. Some sealants will be more effective at sealing for a given volume than others. Some riders may want more liquid sealant left over to deal with punctures. And let’s not even get into how various tire inserts might affect things.
This calculator isn’t designed to address all of those variables, but I’ve tweaked it so that it reliably provides a good starting point no matter what width or diameter of tire you’re running. Feel free to adjust the recommended amount slightly for your particular wants and needs, but you shouldn’t need to go up or down more than about 10%. If you get it right (and this calculator should help you get there), you’ll end up with a fully sealed tubeless tire with enough liquid sealant left over to deal with the occasional puncture or small cut.

I should warn you that Substack frustratingly doesn’t seem to provide a way for me to directly embed this calculator into the post itself, so unfortunately, you’ll have to download it below and then access it in a separate app.
I know, kind of a pain (and super annoying for both of us), but that’s also why I’ve made this post free for everyone to access.
Anyway, without further ado, download the file below, give it a shot, and let me know what you think in the comments. Hopefully you find this helpful.







Ah, the engineer is still in you James. Dusted off integral calculus for that derivation. 0.3mm for the average film thickness, yes? Those approximations seem pretty good to me based on what I dump in.
You’re telling me dumping sealant in until it ‘looks about right’ isn’t the industry accepted method?